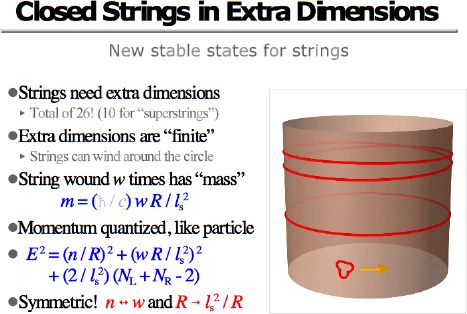
As in our first discussion of extra dimensions, it can be helpful to imagine the analogy of a tightrope. Think of the strings as little rubber bands stuck on the surface of the tightrope (but able to slide freely along it). If they don't wrap all the way around it, they will contract down as close to zero size as their vibrations will allow. But if they do wrap around the circle, they can't contract any smaller than its radius. Stretching around that way carries energy, and as usual the energy will appear in our equations as an effective mass.
As mentioned earlier, string theory only turns out to be self-consistent in a certain number of dimensions. (Technically, there are a ways to formulate the theory with different numbers of extra dimensions or without them entirely, but the alternatives are even less like the observed world. Unfortunately, explaining any of this would take us too far afield.) The 26 dimensions required by "bosonic" string theory include time, so that means 25 dimensions of space instead of the three that we're familiar with. (And superstring theory describes "only" nine dimensions of space.) It is very significant that the theory predicts a number at all! Most theories of physics are either well-defined in any dimension (like general relativity or the standard model) or are specifically designed with a certain dimension in mind. Even though string theory's specific predictions look wrong at first, they are still a step in the right direction.
And as we have seen, the presence of extra dimensions does not immediately rule out the theory: as long as they are small enough, our experiments might not have seen them. To make the meaning of "small" precise, an important first step is to require that they be "finite"—that they don't go on forever like the three dimensions that we normally see. (If they did, we'd almost certainly see them!) In precise mathematical language, a set of extra dimensions that is finite in this sense is called a "compact manifold". We will stick to the simple case where the extra dimensions are circles, though there are many other possibilities.
One important observation is that when closed strings in extra dimensions interact with each other by joining and splitting apart, their "winding number" is conserved. For example, if you start with a string that winds twice around the circle, it could split into two strings that each wind around the circle once, but the total number of windings is still two. (Remember that the strings are "oriented": they know which direction they are wound. So in this example the string could also split into a string wound three times and a string wound "-1" times: once in the other direction.)
As a final technical note, in the equations on the bottom half of this slide I have finally dropped the factors of c and h-bar to reduce clutter. If you keep careful track of units in the equation for the energy spectrum, you can put those constants back in by finding the unique way to make each term have units of "energy2".
The symmetry between momentum level and winding number in this energy spectrum is a surprise, but it turns out to be much more than a coincidence...
Up to my research page.
Up to my professional page.
My personal site is also available.
Any questions or comments? Write to me: jensens@alma.edu
Copyright © 2004 by Steuard Jensen.