An Introduction to String Theory

Slide 30 of 37
<<   [Outline]   >>

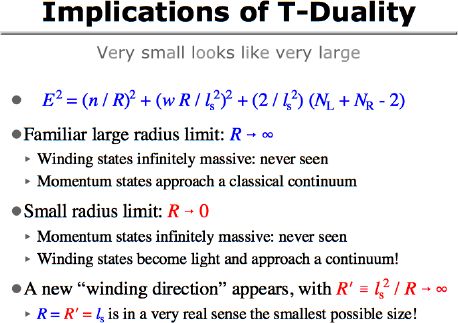
Let's explore T-duality in a little more detail. We start with the energy spectrum for closed strings in extra dimensions from two slides ago. If we look at the limit of large radius as we did before, we see that this gives the same result: the winding states get so heavy that we could never produce them. Meanwhile, the energy difference between adjacent momentum states gets smaller and smaller until it looks like a perfectly smooth, continuous variable.

On the other hand, as R --> 0, winding states act more like our intuitive, continuous notion of momentum than momentum states do! That's the strange reversal of T-duality at work. As the "familiar" dimension of momentum shrinks away to nothing and becomes irrelevant, a new "winding space" dimension seems to appear to take its place.

This is a truly remarkable concept in string theory: there really is a "smallest meaningful size" for a dimension of the universe. And when we get close to that size, our familiar concepts of space and motion begin to completely fall apart. (Building intuition about this odd sort of "winding direction" is part of the motivation for my own current research.)

As a side note, special things happen at the "minimum radius" R = R' = l_s because of the detailed mathematics of the theory, but we don't have time to explore them here. (Just as a taste: when there are "finite" dimensions, the constraint "N_L = N_R" mentioned earlier is generalized to "n w + N_L -  N_R = 0". That means that new "massless" states are allowed, in which only one of N_L or N_R is excited. For example, the state "n = w = 1, N_R = 1" is now allowed, and as can be seen above it has E = 0. Because the total excitation level N = 1, the particle has "spin 1". So these act like a new kind of photon, which leads to interesting effects.)