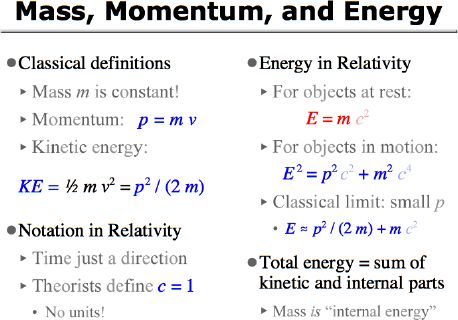
Some of this material is probably familiar to most of this audience, but I'll go through it anyway to get you oriented and to establish the notation that I am going to use later. And it's worth spending a little while to get it straight: there's a lot going on on this slide!
Probably the most important thing to take away from all this is the final point: what we call "mass" is really a measure of how much energy is "bound up" inside a particle where we can't see what's going on. That just sounds like a philosophical point now, but we'll see later on that it can be literally true.
The first section is just a reminder of good ol' Newtonian physics. Mass, momentum, and kinetic energy (energy of motion) are the basic quantities of interest there, and they are related as shown.
Next, we take a step up to Special Relativity, and see how these quantities behave there. First of all, don't fall for the old fashioned perspective that "as things move faster, they get heavier"! Mathematically, that works in some formulas (momentum, for example) but not others (like kinetic energy). The modern perspective on relativity is that "mass" always means "rest mass": a fundamental property of any object.
Now, as I showed in the space-time diagrams earlier, relativity tells us that time is just another direction (although it behaves differently than the usual spatial directions in some formulas). For convenience, theorists generally choose to measure space and time in the same units: in many ways, that just makes sense. (Would you want to measure height in feet and width in centimeters? Didn't think so.)
Because relativity tells us that there is a special maximum speed in nature (which happens to be the speed of light), we can use that speed to convert between our usual units of distance and our usual units of time: we choose units where c is exactly 1. Thus, for example, we can measure both space and time in meters (one meter is a very short time!) or in seconds (one second is a very long distance!). Or, to pick a reasonably familiar example, we can measure time in years and distance in (light-)years. None of those are very convenient units for us in everyday life, but they make a lot of equations much simpler: the special speed c becomes just "1 meter / meter", "1 second / second", or "1 year / year", and we can ignore it completely. I won't do that for a while, though.
Finally, for those wondering where that "classical limit" came from, the explanation isn't too painful if you've heard of a "series expansion". In brief, if a number x is much smaller than 1, the function sqrt(1 + x) is approximately 1 + (x/2). So to find the classical limit of the energy, we just take the square root of both sides and then factor out an mc2. Remaining inside the square root is 1 plus p2/m2c2; formally, this second term is what has to be much less than one in the classical limit. We can then use the square root approximation, and then multiply the mc2 back out. (Ok, so that wasn't really so simple, but hopefully it was enough of a hint that you could try it yourself.)
Up to my research page.
Up to my professional page.
My personal site is also available.
Any questions or comments? Write to me: jensens@alma.edu
Copyright © 2004 by Steuard Jensen.