An Introduction to String Theory

Slide 26 of 37
<<   [Outline]   >>

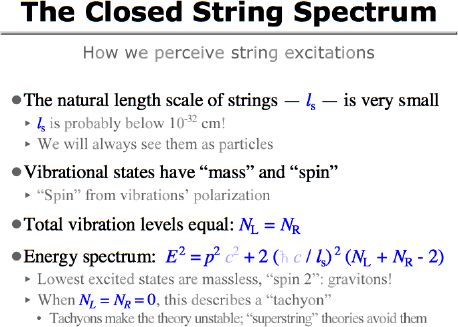
The next step is to figure out what all these excitations look like to us. The short answer is that they'll all look like particles because the strings are so small, but the different vibrational modes will lead to the distinctions between various observed particle types.

The N_L = N_R condition is too complicated to explain here, but in a sense it is necessary in order for the string to come back to the same point after you go around once. (When there are extra dimensions, the condition can change, because there are many physically distinct ways to loop around to the same point.) It might also be possible to think of it as a requirement to have "standing waves": you need equal amounts of wave motion going each way along the string.

The lowest excited states have N_L = N_R = 1, so their effective mass is zero. Technically, these m = 0 states actually include more than just the graviton (the "particle" that carries gravity), but the others aren't important for us at the moment.

("Tachyons" are particles with m² < 0. Why do tachyons "destablize" the theory? Every time one is produced, energy is released instead of being consumed. That means that you could make infinitely many of them and still have (infinitely) more energy for ordinary matter than you had before. Because we don't live in an infinite sea of tachyons, a theory that allows them can't describe the real world.)